
�@Kozo SAITO, Ph.D.
�@Director, Institute of Research for Technology Development (IR4TD) &
�@Tennessee Valley Authority Professor in Mechanical Engineering
�@University of Kentucky
�@Lexington, KY 40508-0503 USA
�@http://www.engr.uky.edu/me/cfpl/
| TED Plaza |
| Impact of "Scale Modeling" on Research and Development -1- |
| Scale Modeling and IR4TD |
|
INTERNATIONAL SYMPOSIA ON SCALE MODELING Scale modeling covers almost all fields of engineering and is often applied to medicine, meteorology, biology etc. Because of its interdisciplinary nature, Professor Emori (the founder of scale modeling theory, known as Law Approach [1]) knew the need for international communication among a wide range of scale modeling researchers. He initiated the first international symposium on scale modeling in 1988 in Tokyo under the sponsorship of the Japan Society of Mechanical Engineers [2]. After the first successful ISSM, unexpectedly, it took nine years to host the second symposium in Lexington, Kentucky under the sponsorship of the University of Kentucky. To keep Professor Emori's will alive, Professors Tadao Takeno and Yuji Nakamura hosted the third ISSM in Nagoya in 2000, and the International Scale Modeling Committee formed just around that time decided to continue ISSM every three years. Based on that agreement, the fourth ISSM was held in Cleveland, Ohio in 2003, hosted by Dr. Vedha Nayagam and Professor Simon Ostrach under the sponsorship of the National Center for Microgravity Research, NASA Glenn Research Center. The fifth ISSM was held in Choshi, Chiba, Japan in 2006, hosted by President Toshi Hirano and Professor Lijing Gao of the Chiba Institute of Science. During ISSM 5, a special workshop was proposed to select papers from the past ISSM and publish them in a special ISSM volume: Progress in Scale Modeling, to be published from Springer in 2008 [3].THE ROLE OF SCALE MODELING Scale models can create scaled down or up versions of the full-scale phenomena. When researchers attempt to properly design prototypes, conduct experiments or observe the scaled phenomena, they may be able to gain at least the following three benefits: (1) Scaling relationships between the prototype and the scale models; (2) Imagination to speculate on the behavior of the prototype phenomena, and (3) Validation of computational model predictions. The third benefit becomes more important in the age of computers. It is no question that computational methods save the time and energy of human calculation, but also provide details in virtual reality conditions under well controlled initial and boundary conditions, that may be difficult to achieve by experiments. However, all computational models require validation. Without validation, no computational models can correctly predict performances of the prototype phenomena. The direct validation of the computational model by prototype tests would be the most ideal, but often faces difficulty due to economical, ethical, or other reasons. The scale model validated by scaling laws can serve as an excellent alternative validation tool for computational models (e.g., Jim Quintiere has proposed the use of reduced scale models to validate numerical simulation of collapse of the 9-11 World Trade Center [3]).AN EXAMPLE OF SCALE MODELING Scale modeling has two different concepts: static similarity and dynamic similarity. Dynamic similarity deals with transient phenomena. You can find more details in reference [1] and web site:http://www.mni.ne.jp/~sekimoto/ScaleModeling.html Here I will give you an example problem of scale modeling. problemIt took 10 minutes to cook a 500g beef in medium rare using a gas grill. Using the same grill with the same temperature set up, how long does it take to cook a 1,000g beef in medium rare? Assume that both beefs are geometrically similar in shape and contents of fat and meet.==> Answer: see Reference [1], pp.38-43. Qk = heat conducted through the beef; Qc = heat accumulated in the beef; c = specific heat of beef; k = thermal conductivity of beef; �� = density of beef; t = time; l = length. All parameters listed are characteristic (representative) parameters which differ from specific parameters (see Reference [1]). Prime (') represents scale models.  �@�@Fig.1 Dynamic similarity  UNIVERSITY OF KENTUCKY'S NEW INSTITUTE: IR4TD The University of Kentucky's new Institute of Research for Technology Development (IR4TD) was proposed in 2006 and approved the next year. IR4TD was created as part of the University of Kentucky's strategy to seek excellence in research, education and service. The mission of IR4TD reads: IR4TD is a newly created research institute dedicated to excellence in engineering research and unique education based on IR4TD-Hitozukuri principles.Two specific aims of IR4TD are: (1) to solve industry's technical problems by developing a win-win working relationship with company's engineers to create new ideas and find value-added solutions which are only possible through collaboration; and (2) to create a learning system which can enhance the process for students, researchers, and visiting scholars through sponsored research projects, helping them to become unique engineers who have ability to function in an ever-changing international arena. It is sometimes claimed that industry and academia are two very different cultures, with the former concerned with how to deliver reasonably-priced high quality products to customers in a timely fashion, while academic institutions focus on education, research, and service [4]. Toyota's Chairman, Mr. Cho stressed the importance of "Hitozukuri culture" during his recent interview in a Japanese TV program (also in his recent lecture at Toyota Motor Vietnam and his related article [5]). A common mission does exist between companies that value "Hitozukuri" and academic institutions that focus on education. Furthermore, at IR4TD, to stress the way research and development are integrated in our work, we call our approach R4D - research for development. The new institute's purpose is to directly and effectively respond to requests from industry. R4D is a demand-pull rather than a supply-push approach; we respond to the needs of clients who approach us rather than approach companies with our research interests. The approach also provides an excellent education for our graduate students in tackling pressing industry problems, working as a team, accepting responsibility, coping with real budgets and real deadlines, communicating effectively with clients, and understanding their point of view. A client may see a problem with the current process; we can see, in fact, that they have come to the limits of a current technology so that a new generation of technology or even a radical new approach to the whole question is needed. The "R" of our work is not simply paired with the "D" but tied directly to needed innovations. Overall, this approach leads to: (1) a high probability of immediate in-plant benefits to the company, and (2) a high likelihood that new technology needs will be accurately identified -- thus, new-generation technologies that fit efficiently into the company's manufacturing systems, and the potential for discovering "quantum leap" (revolutionary) solutions that can be transferred to other industries. In IR4TD, we promote the use of scale modeling through education and research. We created a new course, ME 565 - Scale Modeling in Engineering, a semester long three credit hour course for both undergraduate seniors and graduate students, to introduce them to the concepts and benefits of scale modeling techniques to effectively deliver solutions to industry sponsored projects by observing difficult-to-see prototype-phenomena, highlighting the benefits listed above in (1) and (2). SUMMARY When engineers receive some ideas in new product development, they can test how the new design looks by building scale models and they can get an actual feeling with the prototype through their imagination. The late Professor Emori often said: "When children play with a toy airplane, their mind is wondering about the prototype airplane which they haven't ridden." Children can use the scale model airplane as a means to enter into an imaginative world of wonder by testing, in their own way, how the actual airplane might function, how the actual airplane can maneuver aerodynamically, what might be the actual sound of a jet engine, how to safely land the actual airplane, and so on. This imagination that scale models can provide for children will help them later develop professional intuition. Physical scale models can never be entirely successfully replaced by computer screens where virtual models are displayed and fancy functions are demonstrated. Not only children but also adults can learn things by actually touching things only offered by physical models, helping all of us develop an imagination and a feeling eventually leading toward Kufu [1], a highly developed form of professional intuition. Einstein's famous "thought experiments [6]," which helped him to restructure modern physics may possibly and effectively be taught by letting researchers play with scale models!?Professor Forman Williams wrote Message [7] to JSME about desire and motivation of engineers and researchers, which can constitute the basis of our academic research and education to support this article on scale modeling. I also acknowledge Professor Yuji Nakamura for inviting me to introduce scale modeling in JSME News. References
<<<<<<<<<<<<<<<<<<<<<<<<<<<<<<<<<<< >>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>> �ȉ��C�a��D �ē��F�O�@�i�P���^�b�L�[��w�j �P���^�b�L�[��w�H�w�Z�p�������iIR4TD�j�@���� �e�l�V�[�o���[�I�[�\���e�B�[�i�H�w���@�B�H�w�ȁj��ȋ��� �X�P�[�����f���Ɋւ��鍑�ۃV���|�W�E�� �X�P�[�����f�����O�i�͌^�����j�͍H�w����S�ʂɉ��p�ł���T�O�ł���C ���ɂ͈�w�C�C�ۊw�C�����w�ɂ����p�����D���̊w�ۓI�ȓ�������C �� �]���Y�����i�͌^�������_�̊J��ҁD���ЁuLaw Approach�v[1]�� ���҂Ƃ��ėL���j�͕��L������̊֘A�����҂��W�܂�C���̕������� �L�p���ɂ��ċc�_�������K�v�������������Ă����D�����ō]�狳���́C ���{�@�B�w��̎x���̉���1988�N�ɓ����ɂđ���X�P�[�����f�����O ���ۉ�c�iISSM-1�j���J�Â��� [2]�D����Ɉ�����������iISSM-2�j �͂��ꂩ��X�N��ƂȂ�1998�N�ɃP���^�b�L�[��w�̎x���̉��ŁC�P�� �^�b�L�[�B���L�V���g���ɂĊJ�Â��ꂽ�D�]�狳���̈ӎv�Ɨ��O���p�� ���ׂ��C��O��iISSM-3�j���|�쒉�v�@���É���w�����ƒ����S��@�� �k�C����w�y�������z�X�g�ƂȂ�2000�N�ɖ��É��ŊJ�Â��ꂽ�D��O�� �̊J�Î��ɍ��ېD�ψ���g�D����C�{��c���R�N���ƂɊJ�Â��邱�� ���������ꂽ�D��l��iISSM-4�j�́C�i���K�����ƃI�X�g���b�N���� �i�P�[�X�E�F�X�^����w�j���z�X�g�ƂȂ�CNASA�O���������Z���^�[�� �����g�D�ł��閳�d�͌����Z���^�[�iNational Center for Microgravity Research�j�̎x�����ĊJ�Â��ꂽ�D����������܉�iISSM-5�j�� ��t�Ȋw��w�̕���q�E�w���ƍ��t�Íu�t���z�X�g�ƂȂ蒶�q�s�ŊJ�Â��ꂽ�D ISSM-5�̉�����ɖ{�V���|�W�E���̕��y�����Ɋւ��ċc�_������ʃ��[�N �V���b�v�J�Â���Ă���C�����ł͉ߋ��T��̃V���|�W�E���ōs��ꂽ �u���̒�����I�����ꂽ�_���ɂ��\���������ʍ��FProgress in Scale Modeling[3]�̔��s��ڎw�����Ƃ����肵���D���̓��ʍ���2008.8�� Springer�o�ŎЂ�蔭�s���ꂽ�D �X�P�[�����f�����O�i�͌^�����j�̖��� �X�P�[�����f���i�͌^�j�Ƃ́C���X�P�[���̌��ۂ�������������ȁC �܂��͑傫�ȃX�P�[���ɂ��āi�����\�Ȕ͈͂Łj�Č����邱�Ƃ� �\�ɂ���D�����҂��u���^�i�v���g�^�C�v�j�v��v���悤�Ƃ���ہC �X�P�[���_�E���E�A�b�v�����u�͌^�i�X�P�[�����f���j�v���g���Ď������C �����錻�ۂ𒍈Ӑ[���ώ@���邱�Ƃɂ��C���Ȃ��Ƃ��R�̏d�v�� �m���邱�Ƃ��ł���D���Ȃ킿�C�P�j�u���^�i�v���g�^�C�v�j�v�� �u�͌^�i�X�P�[�����f���j�v�Ƃ̊Ԃ̊֘A���̗����C�Q�j���X�P�[�� �i���^�j�ɂ����Ăǂ̂悤�Ȍ��ۂ��N���Ă���̂���\������z���i�n���j�́C �R�j���l���f���ɂ��\�����ʂ̕]���C�ł���D�R�ڂ̗��_�͌v�Z�@�� ���B�ɔ�������ɏd�v�ƂȂ��Ă���D�������C���l��͂ɂ�茻�ۂ� �������悤�Ƃ���A�v���[�`�͎��Ԃ���ь����Ҏ��g�̃G�l���M�[�� �ŏ����ɂ��邱�Ƃ��ł���Ƃ��������b�g�͂�����̂́C�����Ɂu���z �����Ƃ��ė^����ꂽ�v��������ы��E�������ɂ�����u�[�����ہv�� ���Ƃ��ė^���邽�߁C����������ōČ����邱�Ƃ͋ɂ߂č���ł��� �i�����ۂɋN����Ȃ����Ƃ����Ƃ��ĔF�ߓ���j�D �������Ȃ���C���l���f���͎����ۂ��Č��ł��邱�Ƃ̑Ó��������O�� �]���i������u���v�j���Ă����Ȃ���Ύ����ۗ\���̓���ɂȂ�Ȃ��D ���������Ɛ��l���f���̒��ړI�Ȕ�r�����z�ł��邪�C�l�X�ȗ��R�i�o�ϓI�C �ϗ���̐���Ȃǁj�ɂ���������������ł���ꍇ�C�X�P�[�����f���� ���l���f���̌��Ɏg���邱�Ƃ͓��M�ɒl����i����������C �N�B���e�B�A������9/11�̍��ۖf�ՃZ���^�[�r���̕��ۂɑ��Ė͌^ �����𐔒l���f���̌��Ɏg�����Ƃ��Ă��Ă���j �X�P�[�����f�����O�̈�� �X�P�[�����f�����O���s���ہC�傫���͈ȉ��̂Q�ɑ�ʂ����D ��͐ÓI�������Ƃ��Ēm������̂ŁC������͓��I�������� �Ă����̂ł���D��ҁi���I�������j�ł͔���_�C�i�~�b�N �V�X�e���������D�����̏ڍׂɂ��Ă͕����P���邢�͈ȉ��� �E�F�b�u�T�C�g�ŏЉ��Ă���̂ŋ�������l�͎Q�Ɗ肢�����D http://www.mni.ne.jp/~sekimoto/ScaleModeling.html �T�^�I�ȃX�P�[�����f���̗��p��������ŏЉ�悤�D �y��z�K�X�R�����ɂ�500g�̓����~�f�B�A�����A�ɗ�������̂�10�� �K�v�ł������Ƃ���D�����R������p����1000g�̓����悤�ɒ��� ���悤�Ƃ����牽��������ł��낤���H�������C�d���̈قȂ���͊� ���w�I�ɑ����ł���Ƃ��C����鐬���i�����Ȃǁj�����������ł���Ƃ���D Qk�F����ʂ��ē`���M�CQc�F�������Œ~�ς����M�C���F���̔�M�C k�F���̔M�`�����C�ρF���̖��x�Ct�F���ԁCl�F�����Ƃ���D������ �������S�Ẵp�����[�^�͌n�̓����l�i��\�l�j�ł���C����i�n���L�ȁj �p�����[�^�ł͂Ȃ��i�ڍׂ͕����P���Q�Ƃ̂��Ɓj�D�܂��C�f�͖͌^ �i�X�P�[�����f���j�̏��ʂ�\���D �i���ɂ��Ă͌����Q�Ƃ̂��Ɓj �P���^�b�L�[��w�ɂ�����V�������݁FIR4TD �P���^�b�L�[��w�ł́C2006�N�ɐV�����t�u�{�݂Ƃ��āu�H�w�Z�p������ �iInstitute of Research for Technology Development�GIR4TD�j�v�� �J�݂��Ă��C���N���̐ݒu���F�߂�ꂽ�DIR4TD�́C�����C����C����� �T�[�r�X���s�����߂ɐV�������@��͍����Ă���P���^�b�L�[��w�̐헪 �̈�ł�����D�����ł͑�z�����H�w���������{�����ł���Ɠ����ɁC �u�l����̗��O�v�Ƃ������قȋ���R���Z�v�g���~�b�V�����Ƃ��Čf���Ă���D ��̓I�ȖړI�͉��L�̂Q�_�ł���D �P�j�@��Ƃ̋Z�p�I�Ȗ����C��Ƃ̋Z�p�҂Ɨ͂����킹�ĉ������邱�Ƃ� �u���ݏ����iwin-win�j�v��D�����ł����uwin-win�v�W�Ƃ́C���� ������ʂ��ď��߂ē�����t�����l�̂���������V�����A�C�f�B�A�� �n���������炷���Ƃ��w���i��ƂɂƂ��Ă͊w�p�x�[�X�Ŕ|��ꂽ������ �Ƃ��������b�g���C��w�ɂ����Ă͎��ۂ̖�������ʂ��Č����Ҏ��g�� ���炳���Ƃ��������b�g���ɓ�����̂ł���j�D �Q�j�@�w���C�����ҁC�C�O���ٌ����҂��C�i�ʏ�̊�Ɠ����̂悤�Ɂj�X�|���T�[ �̂����v���W�F�N�g�`�[���ɓ���C�ӔC���闧��ɂČ��������ɏ]�������� ���ƂŁC�u���n�g���[�j���O�iOJT�j�v�ɋ߂��V�X�e���ɂ��ނ玩�g�̋Z�p�� �Ƃ��Ă̔\�͂��J�Ԃ����C�ǂ̕���ł��ʗp�������͈͂̍L���l�ވ琬������ �Ƃ������u�i����܂łɂȂ��j�Ǝ��̋���V�X�e���v�����グ�邱�� ���ɁC��ƂƑ�w�Ƃ͕������Ⴄ�̂Ő[���Ƃ���܂ŗ����������Ȃ��Ƃ��� �ӌ����悭�����D�m���ɑO�҂͔[�������C�����ō��i���Ȑ��i���^�C�����[ �Ɍڋq�ɒ��邱�Ƃ��g���ł���̂ɑ��C��҂́i�Z���Ԃł͐��ʂ��킩 ��Ȃ��j����C�����C�T�[�r�X����邱�Ƃ��g���ł��邩��ł���[4]�D �������Ȃ���C�g���^�����Ԃ̒��x�m�v��͍ŋߕ��ꂽ���{�̃e���r �ԑg�̓��W�ɂāu�l���蕶���v�̏d�v���ɂ��ċ������Ă���i������|�� ���e���g���^�̃x�g�i���x�Ђł̍u���C���邢�͊֘A�}��[5]�ɂĉ{���ł���j�D �܂�C�ꌩ�u�����荇��Ȃ��v�Ǝv�����ƂƑ�w�Ƃ́C�u�l����v�� �����Ӗ��ł͋��ʂ̗��O���������킹�Ă���̂ł���D �����IR4TD�ł́C�u�����iResearch�j�v�Ɓu�J���iDevelopment�j�v�������� �u�����Ȃ��v���Ƃ��d�����Ă���D���̂��Ƃ�R4D�A�v���[�`�i�J���̂��߂� �����FResearch for Development�j�ƌĂԁD��X�̌������͈˗���ł����� �̗v���ɑ��Ē��ړI�������I�ɑΉ�����DR4D�Ƃ́u�����ґ����牟���t�� ��v�̂ł͂Ȃ��C�u���v����������o���v���@�ł���D ��������C��X�̌����Ɋ�Ƃ��A�v���[�`�u������v�̂ł͂Ȃ��C��X�� �A�v���[�`���Ă�����Ƃ̗v���Ɂu������v�Ƃ������Ƃł���D �܂����̕��j�ł́C��w�@���B���Y�ƊE�ً̋}�ۑ���`�[���Ǝ��g�݁C ���ۂ̐ӔC�������C���ۂ̗\�Z��[���ɂ��Ĉ˗���i�N���C�A���g�j�� �����I�Ɉӎv�a�ʂ��s���C���̉ۑ�̍����𗝉�����Ƃ����悤�ȁC��ʂ̋��� �Ƃ͈�������炵���u�i�l�ށj����v�̋@������D �˗���͌���Z�p�ɑ��āi����T�C�h����j��������̖��������Ă���D ��X�͂��������Z�p�́u�ǁv�Ƃ��ĕ߂炦�C������Z�p�̓������邢�͑S�� �̖��ɑ��Č��I�ȁu�ω��v�ɂ��������邱�Ƃ����݂�D���̐V�������z �ނ��߂ɁgR(����)�h�����������D ���̂Ƃ�R��D�i�J���j�Ə�Ƀy�A�ł��邾���łȂ��C�K�v�Ƃ����V�����Z�p �ɒ��ڊ�^������̂ɂȂ�D ��������ƁC��X�̌f���邱�̐V�������j�ł́C���̂悤�ȉ\��������D ���Ȃ킿�C�P�j�����\�������鎩�H��̑����I�ȗ��v�̌���C�Q�j�V���� �Z�p�̃j�[�Y�����m�ɔF������鍂���\���C���Ȃ킿��Ƃ̐��Y�V�X�e���� �����I�ɑg�ݍ��܂��V����Z�p�Ƒ��̎Y�Ƃɂ��ړ]�\�ȁu����I�Ȑi�� �iquantum leap�j�v���i�v�V�I�ȁj������̑n���C�ł���D ���̎����̂��߂ɁCIR4TD�ł͌�������ы����ʂ��ăX�P�[�����f���i�͌^�����j �̊T�O�𗘗p���邱�Ƃ�ϋɓI�Ɋ��߂Ă���D����V�X�e���Ƃ��ẮC�V���� �J���L�������ɂ����Ė͌^�����Ɋւ���u�`�iME565-Scale Modeling in Engineering-�j ���J�u���C�R�P�ʕ��̏[�������u�`�Ƃ��Ă���i�Ώۂ͊w���S�N�C��w�@���j�D �����ł́C�X�P�[�����f�����O�̊T�O�i��b�j�Ɖ��p������炷�邱�ƂŁC ���X�P�[���ł́u�����Ȃ��i���邢�͊ώ@���ɂ����j�v�ʂ�͌^�����ɂ�� ���݉������Ă��ǂ��������C�e�l������L�v���W�F�N�g�̉𖾂𑣐i ������̂Ɋ�^����D����ɂ���L�ŋ������P�j�ƂQ�j�̎����������炷�D �܂Ƃ� �G���W�j�A�i�Z�p�ҁj�͐V�������i�̊J�����ɂ������āC���ꂪ�ǂ̂悤�� ������̂���͌^����邱�Ƃɂ���Ċm�F���C���ۂ̐��i�̃C���[�W�� �u�i���A���Ɂj�z���v����D�͌^�������_�̑n�n�҂ł���̍]�狳���� �����̒��ł����q�ׂĂ���F�g�q������s�@�̂�������ŗV�ԂƂ��C �ނ�̒��ł́u�܂���������Ƃ̂Ȃ��v��s�@���ǂ�Ȃ��̂��v���`�� �Ȃ���V�ԁh�ƁD�q���͖͌^�i�X�P�[�����f���j�ł����s�@�̂������� ���g���āC�ނ�̓Ǝ��̕��@�ɂ��z���̐��E�ւƏ��҂���@ �|�{���̔�s�@�ł͉����ǂ����삵�C���c����̂��C�ǂ�����Ĕ�s����̂��C ���ۂ̃G���W���͂ǂ�ȉ�������̂��C�ǂ�����Ĉ��S�ɒ�������̂��|�D �͌^�������炷���́u�z���́v�́C�����ނ�̐�啪��ɂ����āu�����I �ȓ��@�͂̔��W�v�ɖ𗧂D�͌^�Ƃ͂����C��ŐG�芴���邱�Ƃ��ł��� �u�����v�͐��l���f�����g���ē�����X�N���[���ɉf���o������ �摜�i���Ƃ����ꂪ���A���ɒ��F����Ă��悤���C���낢��ȕ����ʂ�\�� �ł���@�\�����Ă��悤���j�Ƃ͔�r�ɂȂ�Ȃ��قǂ̑z���͂����߂Ă����D ���̂��Ƃ́C�q���Ɍ��炸��l�ł������ł���D�u�����v�ɐG��邱�Ƃ� �z���͂����������C�Ђ��Ă͌��ʓI�ȁu�H�v�|�v���Ƃ��Ă̒��ϗ́|�v���D �ߑ㕨���w�ɉ��v�������炵���A�C���V���^�C���̗L���ȁg�v�l����[6]�h�Ƃ́C �Ƃ���Ȃ������C�͌^���g���ĕ������u�z���v���錤���҂Ɠ������Ƃ�������Ȃ��D �M�҂̉��t�ł���C�R�ė��_�̐��E�I���Ђł���E�B���A���X���� �i�J���t�H���j�A��w�T���f�B�G�S�Z�j�́C�ߋ��ɓ��{�@�B�w��w�Ȉ��� ���������b�Z�[�W�̒��ŁC�G���W�j�A����ь����҂̌����ӗ~�Ƃ���� �x���铮�@�̏d�v�����������Ă��� [7]�D�E�B���A���X�����̍����� �ȑf�Ȍ����ҁE����҂Ƃ��Ă̎v�z�́C�M�҂̃X�P�[�����f�����O����� �����̊�b�ƂȂ��Ă���D �Ō�ɁC�͌^�����̊T�O���M�w��M�H�w����̉���e�ʂɏЉ��@��� �^���Ă��ꂽ�����S��k�C����w�y�����Ɋ��ӂ��C�M���Ƃ������D �y��ҁF�����S��i�k�C����w�j�z |
| TED Plaza |
| Impact of "Scale Modeling" on Research and Development -2- |
| �֓���k�Ђɂ�����핞�ƐՉА����̖͌^���� |
|
�P�D�͂��߂� �@ �@1923�N9��1���C�֓���k�Ў��ɓ��������̗��R�핞�ƐՂŋN�������А����́C 15���]�̊Ԃ�38,000�l�̖���D�����Ƃ�����S���ł������D ��n�ł������핞�ƐՂɂ͉Ђ��瓦��悤�Ƃ�����4���l�ƁC�ꕔ�̐l���^�� ���ꂽ�ƍ������7���u�̕~�n�ɂ��ӂ�C�ߌ�4���C��w�̐��������̍L��� ���W���l�n�Ɖƍ�����̉R���������グ�C�����������͓����f���� �̓���ɗe�͖����~�蒍�����D�����c�����҂͂킸��5����2,000�l���炸�ł������D�@���̂悤�ȍЊQ�̔������J�j�Y����m�鎖�́C�h�Џ���ɏd�v�Ȏ��ł���ɂ� ������炸�C�ЂȂǂ̑�K�͍ЊQ��������Ŏ������鎖�́C������s�\�ł���D ��r�I���K�͂ɏk�����čČ��ł���Ȃ�C�����͂͂邩�ɗe�ՂɂȂ�C ���̎������ʂ������ɂ܂œK�p�ł���C���ۂ̖h�Б����Ăɐ��������Ƃ��ł���D ���݂܂łɍs��ꂽ�А����̖͌^�����ɂ��ďЉ�����D |
|
�Q�D���n���̉А����͌^���� �@ �@1970�N��ɋC�ی������̑��n���ɂ���ĉА����̌n�����Ă��������n�߂�ꂽ�D TV�j���[�X�ő��n�����s�����А����̖͌^�����̕�ɐڂ��C�������t�ł��鐬����w�����@ �]���Y�搶�̉��Ŗ͌^�����ɂ��Ċw��ł������́C��y�̐ē��F�O�� �i���P���^�b�L�[��w�@�B�Ȏ�ȋ����j�ƁC�����ÁX�ł��̎����ɂ��Č�荇���� �����͂�����o���Ă���D ���̑��n���̌�����ē������p���C�����������`�����ɂȂ낤�Ƃ͖��ɂ��v��Ȃ������D�@�핞�ƐՂ̉А����ɂ��ẮC�]���O���ʉ߂ɔ����ǒn���������ł͂Ȃ����� �l�����Ă�����[1]�C���n���͉����ƉЂɂ���ċN����㏸�C���Ƃ̑��ݍ�p �ł͂Ȃ����ƍl���C�͌^�����ɂ���Ă����������[2]�D �Ў��ɔ������闳���͂������̌^���ώ@����Ă���C�����ƍL��Ђ̑��� ���ʂƂ��Ĕ�������А������C���n���͔핞�ƐՌ^�ƕ��ނ��C��X������ɏK�����ɂ���D �@�����ɂ�����k��1/2500�̖͌^����[3]�ɂ��핞�ƐՌ^�А����̔����ɐ������� ���n���́C1979�N11�������p�����n�ɂ����đ�K�͂ȉА����̎������w�����C �ē���������ɉ�������D �А����������̗����핞�ƐՂ��X�P�[���_�E���������̂ŁC1/100�ɂ��Ă��R�� �G���A�̈�ӂ�25m�ɂ��y�ԑ�K�͂ȕ��ł���D ���n���́C���ۂ��N�����G���A�̑�����ɍ��킹�����̕����𐄒肵�C ���R�����ł��̏�����������������I�ю������s����[3][4]�D �@�z���苭�߂ł��������R������܂�n�߂����C�������̉����₩�ɉ�]���n�߁C �����₩�ȐԐF���ɗ���҂��ׂĕ�R�Ƃ��̐�����������C���Y�ꂽ�B�e�v�� �͈ꖇ�̎ʐ^���c���Ȃ������ƌ����i�����ɎQ�������ē����k�j�D �ȗ�25�N�]�C�핞�ƐՌ^�А����̐�����́C���̒m����茩���Ȃ��D |
|
�R�D���z�������Е����ɂ�������� �@ �@2005�N11�����C���{�E�ǔ�TV��萳���̓��ʔԑg�ŕ�������������C ���}�А����̎������ł��Ȃ����ƓˑR�̈˗����������D �ǂ̔M�ӂɕ����ē����Ȃ�тɃP���^�b�L�[��w�̌K�����i������y�����j�� �N���X�}�X�x�ɂ��L�����Z�����C�N�������l�܂���27���}篗��������D �@12��28�����̌��z�������Ƌ����œ����Е����������ɉ����� �������J�n���ꂽ�D�O�C�z�����G�b�t�F���^�Е����́C�S��62m�C �������̑S��15m�C��5m�C����5m�C�䍑����̉Зp�����ł���D�@��������ځC���n���̎����ɏK���������蕔�ɐ}�P�̂悤��L���^�ɔR���� �w�v�^���𒍓�����p�^�p����z�u�����D L���Z�ӑ���ɂ��ĔR�Ă����C���x���z�u��ύX�����Ƃ���CL�����ӑ������� ����3m�ȏ�ɒB���鐷��ȉ��̐��������I�ɏo������Ɏ������i�}�Q�j�D ����L���z�u�͔핞�ƐՂ�͂������ł���C�L��̓�ӂ���Ђ������Ă��� ��P�������Ă���D |
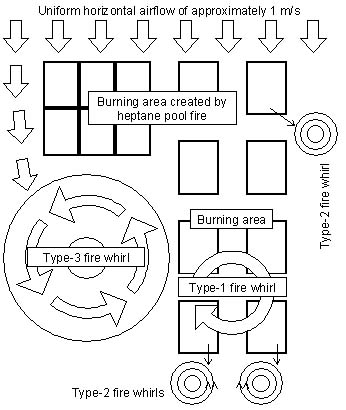 �@�@�}�P�@�R�Ĉ�i�p�^�p��14�ӏ��j�ƉА����̔����ꏊ  �@�@�}�Q�@����3���ȏ�ɒB�����А��� |
|
�Ƃ��낪�핞�ƐՂł͍L���������삯������ƋL�^����Ă���CL�����ӑ���
����ȉА����i�}�P�@Type-1,2�j���������Ă��CL���������ɐ�������������
����Δ핞�ƐՂ̍Č��Ƃ͌����Ȃ��D
���̓��C�������v�O�Ɏ������I�����Ă����Ȃ�C��̈łƊ��C�̏�����ꂸ�C
�d��Ȍ��ۂ����߂����Ă��������m��Ȃ��D��p�̂��߃����K�ʂɎT��������
���C�ƂȂ�CL���������ʼn�]���Ă���i�}�P�@Type-3�j���ƂɋC�t�����̂�
�ق�̋��R�������D��X�͉₩�ɐF�߂��C�ԍڗp���������ĉΉ����ȊO��
��C�����N���ɂ��鎖�����݂��D
�@�}�R��L���������ɏo�����Ή���Ȃ��А����ł���D TV�ǃX�^�b�t���f���ɃC���p�N�g���������邽�߁C�������ɖؕЂ�V�����b�_�[ �_�X�g��u���ƁC�ȒP�ɂ����Ɉ����C�R���Ȃ���Q�Ɋ������܂ꂽ�i�}�S�j�D �핞�ƐՂŋN�������l�n�C�ƍ�����R���Ȃ���Q�ɋz�����܂�C�V��ɕ��� �オ�����Ƃ���錻�ۂ́C���ɍČ����ꂽ���ۂɑΉ�����ƍl���ėǂ����낤�D |

 �@�@���F�}�R�@�R�Ĉ�O�ɏo����������Ȃ��А��� �@�@�E�F�}�S�@�R�Ĉ�O�ɏo�����C�����������А��� |
|
�S�D������ �@ �@�͌^�������s���ꍇ�C���^�Ɩ͌^�̑Ή������Ă��Ȃ���Ύ����͒P�Ȃ�萫�I�� �����ɏI����Ă��܂��D�����Ō����Ή��Ƃ͊w�I�ɑ����ł��邱�Ƃ͌����ɋy���C ���̗͊w�C�G�l���M���x�C���w�����̊e�ߒ��ł��Ή������C�͌^�̎������ʂ��� ���^�̏�Ԃ�c�����邱�Ƃ����߂���D �@���������ɂ͌��ۂɊւ��́C���邢�͔M�̂悤�ȓ���̕����ʂ̔�i�p�C�i���o�[�j �����߁C���̔�����^�C�͌^�ň�v����悤�Ɏ������s���Η͊w�I�ɑ����Ȏ����ƂȂ�D �������̓��o�ɂ��ẮC�����T���Q�Ɗ肢�����D�А����ɍ�p���Ă��镨���@���̗͂ɂ��čl����ƁC�����ƉΉ��ɂ��㏸�C���̊����́C �M����ꂽ��C���镂�͂��x�z�I�ɍ�p���Ă���ƍl�����C���͂͏d�͂ɂ���p �ł��邩��C�����͂Əd�͂̔�C�t���[�h����������D |
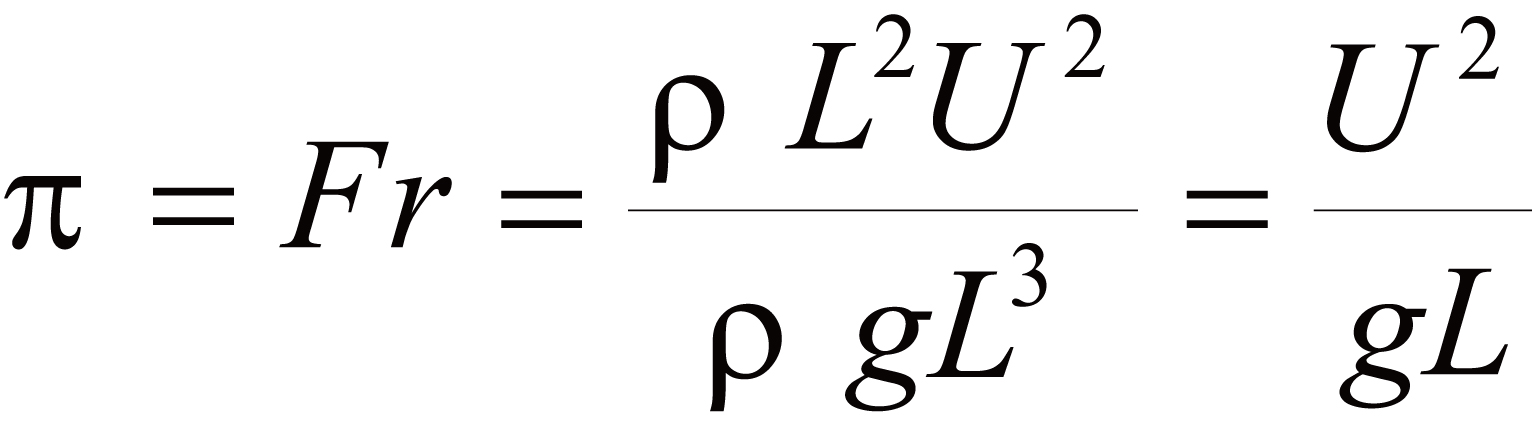 �ρF���x�CL�F�����CU�F���x�C���F�d�͉����x |
|
�@�M�ɂ��čl����C�R������̔����M�ʁC���x�㏸���ċC�̂ɒ~�ς����M�ʁC
���x�㏸���ĔR���\�ʂɒ~�ς����M�ʁC�R���̋C�����M�C�Ή��\�ʂ̍����w����
�̕��˔M�ʂȂǂ��e�����Ă���ƍl������D
�����܂̔M����l�̃p�C�i���o�[���K�肳��邪�C�������^�C�͌^�œ���R����
�g�p����Ȃ�C���_���������Ƃ����l�̃p�C�i���o�[�͎����I�Ɉ�v����[5]�D
�ȏ�̂��Ƃ����{�I�ɉА����̓t���[�h���x�z�ɂ�錻�ۂƍl���ėǂ��̂ł��邪�C ���ۂ̉Ђ��ؑ��Ɖ����̔R�Ăł���̂ɑ��C�����ł͉t�̔R�����g�p���Ă���D ���̎����̉Ή�����3m�����̂܂܊w�I���������ɓ��Ă͂߂�ƁC���ۂ̉Ή������� 3,000m�ɂ��Ȃ��Ă��܂����߁C�Ή������ɒ��ڂ����V���ȑ�������������Ă���[7]�D ���^�Ɩ͌^�̑Ή������Ă��鎖���m�F����͔̂��ɏd�v�ȍ�ƂŁC���ꂪ�ł���� �͌^���猴�^�̌��ۂ𐄒肵�ėǂ��ƌ������ɂȂ�D �@�������̓��o�͈ꌩ�ȒP�Ɍ����邪�C�P���Ȗ���ߋ��ɗޗ���o�����Ă���ꍇ�������C ���߂ɓ����������������̂܂ܓK�p�ł�����͂��܂薳���D �А����̗�ŁC������C�̔S���͂����ۂɐ[���֗^���Ă���ƍl����Ȃ�C �S���͂Ɗ����͂̔�ł��郌�C�m���Y���ƃt���[�h���x�z�̌��ۂƂȂ�C���ꗬ�̂ŗ��� �������邱�Ƃ͏o�����C�͌^�����͕s�\�ƂȂ�D ���̂悤�Ɍ��ۂ��x�z���Ă��镨���@�������ɂ߂��Ƃ́C�{�����\���������Ă��邱�Ƃ� �v�������D ��X�͐V���ɐ��삵���S��4m�C���蕔�̕�0.6m�C����0.5m�̏��^�G�b�t�F���^�R�Ď����p�����ŁC ���݉А����̖͌^�����𑱂��Ă���i�}�T�j�D ������0.1m/s�܂ŗ��Ƃ�����̂́C���̒��x�܂ŕ����𗎂Ƃ��Ƌ�C�̔S���̉e�������� �ł��Ȃ��Ȃ�C�А��������ۂƓ���������Ŏ������邽�߂ɂ�������ɂ͎����ƌ��E������C ����̕]�����K�v�ɂȂ邽�߁C�V���ȍH�v���������̊m���ɋ��߂��Ă���D �͌^������͕���8,9���Q�Ƃ��Ă������������D |
 �@�@�}�T�@���^�����ōČ������А����i��^�����ɑΉ����������ɏo���j |
|
�T�D�܂Ƃ� �@ �@�����ɂ�����А����̖͌^�����ɂ���āC�핞�ƐՂŋN�������А����̃��J�j�Y���� ����ɖ��炩�ɂȂ��Ă��Ă���D�������C���Ȃ��S�̑��������Ă��Ă���킯�ł͂Ȃ��D ���Ƃ��C�А��������c���n�͂��đΊ݂��P�������Ƃ̌���[10]������C ���̐^�U�͍������ĉ���Ȃ��D ���Ȃ��Ƃ���X���s���������ł́C�ߖT�ʼnΉ��Ɉ������܂��А����ȊO�C����Ɉړ����� ���̂͑S���ώ@����Ă��Ȃ��D�����̊ϑ��f�[�^���琄�ʂ��ꂽ�핞�ƐՂł̕����C������ �{���ɐ������̂��C�А����͂ǂ��Ŕ������āC�ǂ̂悤�ɓ���������̂����C�^��͐s���Ȃ��D�@��A�̖͌^�����ɂ�茻�ۉ𖾂̎����͒͂߂��D����̌����ɂ�蔭�����₷���������i�荞�݁C ����А������N�������Ȃ����������o���čs����C�핞�ƐՂŖS���Ȃ����]���҂̑����� �ʂɂ��邱�ƂȂ��C����̖h�ЂɕK�����������̂Ɗm�M���Ă���D |
|
�Q�l����
|